Hey guys, we have started not long ago to learn the term of limes.
So the known definition of the limit of a series goes like that:
If
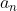 converges to 0, then
converges to 0, then
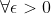 , there exists an
, there exists an
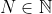 s.t.
s.t.
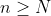
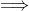

I have some elementary questions about the definition, would it be correct if I use the definition a little bit? such that:
* If
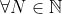
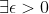 s.t.
s.t.
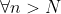 we get
we get
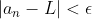
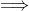

* If there exists
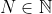 s.t.
s.t.
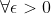
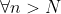 we get
we get
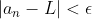
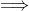

And another type of limes question:
If the series
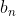 is defined by
is defined by
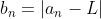 and converges to 0 then
and converges to 0 then
 ?
?
Thank you!
So the known definition of the limit of a series goes like that:
If
I have some elementary questions about the definition, would it be correct if I use the definition a little bit? such that:
* If
* If there exists
And another type of limes question:
If the series
Thank you!
