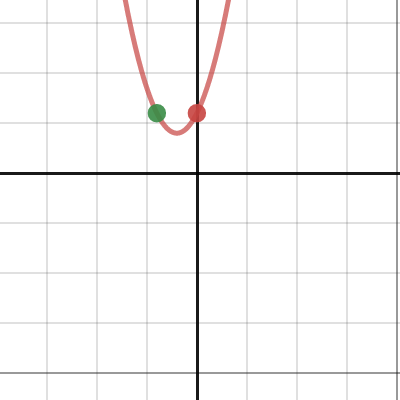
I'm having trouble understanding what effect the middle term ([MATH]2x[/MATH]) in the function below has on the graph:
[MATH]f(x) = x^2 + 2x + 1[/MATH]
I'm trying to intuitively understand. I have a good grasp on the [MATH]x^2[/MATH] graph and also the [MATH]+1[/MATH] shift and can draw the graph [MATH]f(x) = x^2 + 1[/MATH] just by looking at the equation, but I can never intuitively grasp where the graph will end up when the middle term is included. Any help in understanding is much appreciated.
Note: I know what the graph looks like and I'm not just interested in this specific equation, but more the intuition behind the shift the [MATH]2x[/MATH]/middle term causes.
[MATH]f(x) = x^2 + 2x + 1[/MATH]
I'm trying to intuitively understand. I have a good grasp on the [MATH]x^2[/MATH] graph and also the [MATH]+1[/MATH] shift and can draw the graph [MATH]f(x) = x^2 + 1[/MATH] just by looking at the equation, but I can never intuitively grasp where the graph will end up when the middle term is included. Any help in understanding is much appreciated.
Note: I know what the graph looks like and I'm not just interested in this specific equation, but more the intuition behind the shift the [MATH]2x[/MATH]/middle term causes.