The following text says that current density variation induces azimuthal B and axial E.
1. How this statement is explained (I know that current carrying wire induce B around it)?
2. How the Maxwell equations and cylindrical coordinates are used to give d(rB)/dr=rj(r,t) and dE/dr=dB/dt?
3. How B and E from these two equations are used in the motion equation below to obtain the particle energy?
"The text is:
Axisymmetric, cylindrical current distribution j(r, t) which is finite in thickness and initially annular in shape. The distribution contracts rapidly to the axis. Such a time variation in the current density gives rise to both an azimuthal magnetic field By(r, t) and an axial electric field E(r, t) whose values are derived from Maxwell’s equations:
d(rB)/dr=rj(r,t)
dE/dr=dB/dt
The equation of motion in two dimensions is
mr’’ = -ez’B (r, t),
mz’’ = er’B (r, t) +eE(r, t)
where m is the ion mass, e is the ionic charge, and r’ and z’ are the velocity components.
Maxwell equations:
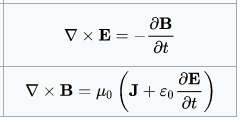
Cylindrical coordinates:
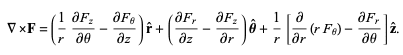 "
"
1. How this statement is explained (I know that current carrying wire induce B around it)?
2. How the Maxwell equations and cylindrical coordinates are used to give d(rB)/dr=rj(r,t) and dE/dr=dB/dt?
3. How B and E from these two equations are used in the motion equation below to obtain the particle energy?
"The text is:
Axisymmetric, cylindrical current distribution j(r, t) which is finite in thickness and initially annular in shape. The distribution contracts rapidly to the axis. Such a time variation in the current density gives rise to both an azimuthal magnetic field By(r, t) and an axial electric field E(r, t) whose values are derived from Maxwell’s equations:
d(rB)/dr=rj(r,t)
dE/dr=dB/dt
The equation of motion in two dimensions is
mr’’ = -ez’B (r, t),
mz’’ = er’B (r, t) +eE(r, t)
where m is the ion mass, e is the ionic charge, and r’ and z’ are the velocity components.
Maxwell equations:
Cylindrical coordinates:
