I have looked extensively for a solution to the below defined problem, and have found none. Let me save "you" time: I am NOT seeking the answer to "how many balls can I fit in a cylinder". I am well aware of the "64 to 74%" rules of thumb. Neither of those 'solutions' solve my problem.
The problem stated as clearly as I can is:
1) I have a well-defined, uniform, unvarying volume. (I need to increase the WEIGHT of a cylinder.)
2) I need to add as much weight to that cylinder as I can using spheres of pure lead. (RhoPb = 11.34g/cc)
3) It is obvious that the smaller the sphere I use, the higher the bulk density will be. However, there are practical reasons (handling problems) for not using spheres smaller than a given size.
4) The 'handling problem' does not have a "threshold". Meaning that regardless of the 'starting' sphere diameter, as sphere diameter gets smaller, the handling problems increase.
5) The 'issue' is that I want to maximize the weight of the cylinder, but I don't want to have to deal with the handling problems associated with "small" spheres. In other words there is a point of diminishing returns, where whatever gain is realized in increased weight is offset by handling issues.
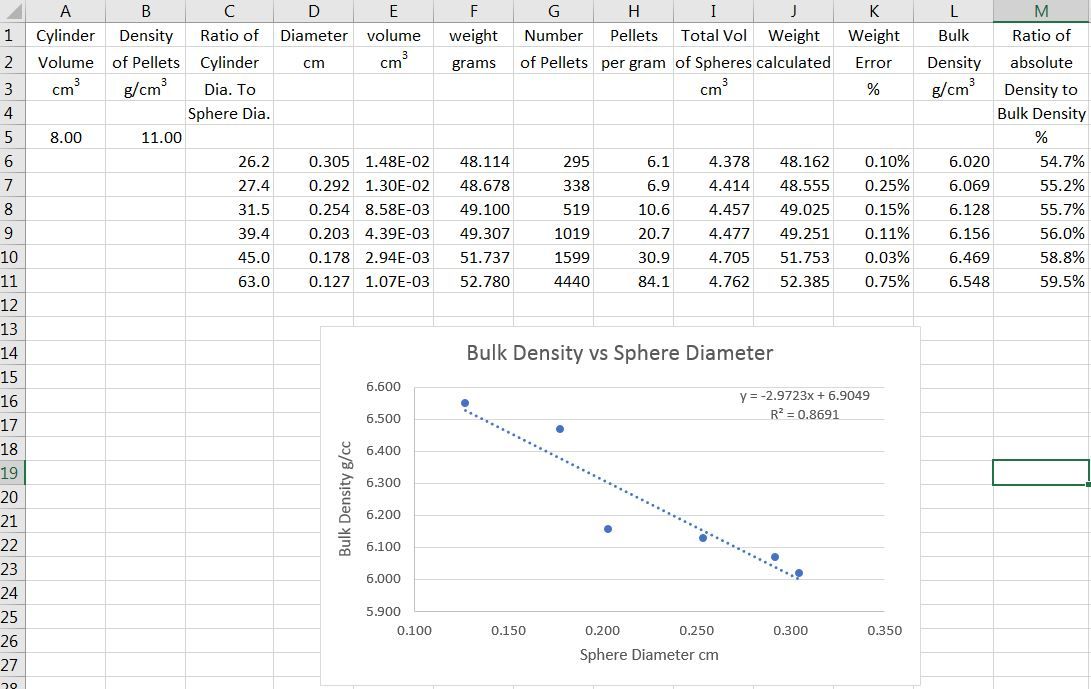
I want to be able to calculate BULK density in a FIXED SIZE cylinder as a function of sphere DIAMETER when sphere DENSITY is constant. The cylinder diameter and height are >> than the largest sphere diameter.
Let me restate the problem in "simple" language:
How does sphere diameter affect bulk density in a given volume?
Thanks!
Paul
The problem stated as clearly as I can is:
1) I have a well-defined, uniform, unvarying volume. (I need to increase the WEIGHT of a cylinder.)
2) I need to add as much weight to that cylinder as I can using spheres of pure lead. (RhoPb = 11.34g/cc)
3) It is obvious that the smaller the sphere I use, the higher the bulk density will be. However, there are practical reasons (handling problems) for not using spheres smaller than a given size.
4) The 'handling problem' does not have a "threshold". Meaning that regardless of the 'starting' sphere diameter, as sphere diameter gets smaller, the handling problems increase.
5) The 'issue' is that I want to maximize the weight of the cylinder, but I don't want to have to deal with the handling problems associated with "small" spheres. In other words there is a point of diminishing returns, where whatever gain is realized in increased weight is offset by handling issues.
I want to be able to calculate BULK density in a FIXED SIZE cylinder as a function of sphere DIAMETER when sphere DENSITY is constant. The cylinder diameter and height are >> than the largest sphere diameter.
Let me restate the problem in "simple" language:
How does sphere diameter affect bulk density in a given volume?
Thanks!
Paul