Cosine law with quadratic equation

[bed]

Hi, I need some help with this question. I have to solve for x using the cosine law. Thank you!

 

[Deleted member 4993]

Hi, I need some help with this question. I have to solve for x using the cosine law. Thank you!
View attachment 33498

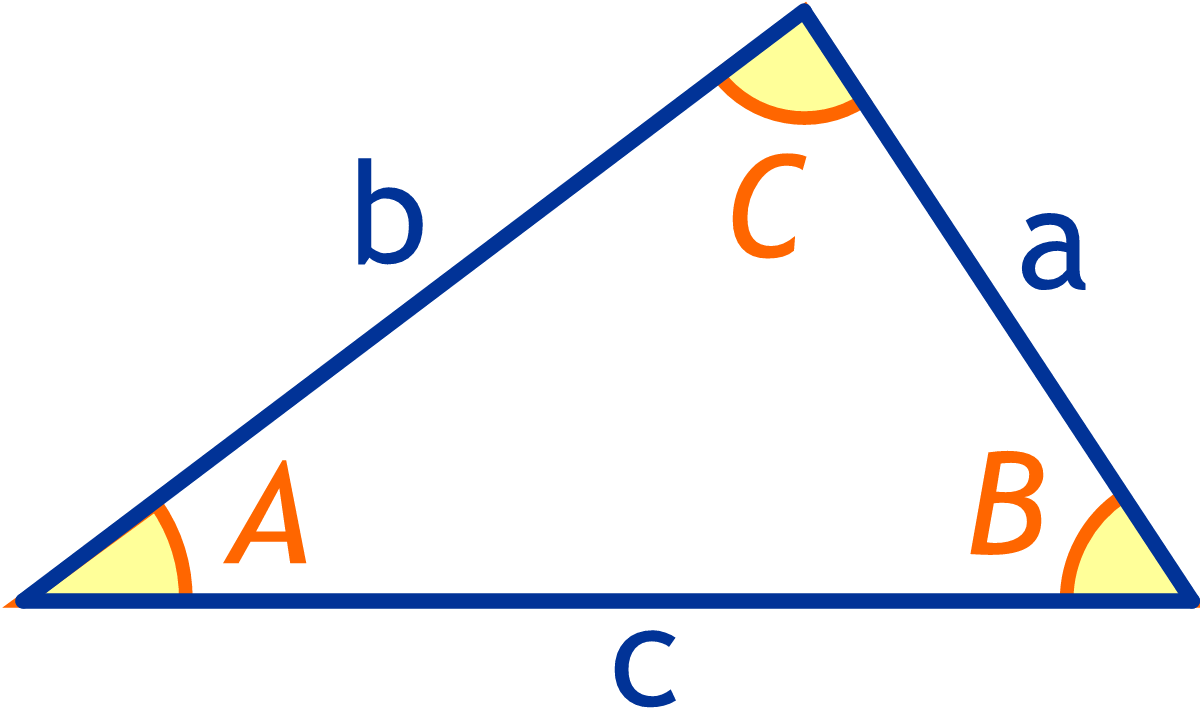
Do you know the laws of cosines for a planar triangle?

Please show us what you have tried and exactly where you are stuck.

Please follow the rules of posting in this forum, as enunciated at:

READ BEFORE POSTING​

Please share your work/thoughts about this problem

 

[Otis]

Hi bed. Here's a link to a web page that explains the Law of Cosines and shows worked examples of how to use it. Your exercise may be solved the same way. Please share how far you can get, or tell us which part(s) you're unsure about, and we can go from there.

The Law of Cosines

For any triangle ... a, b and c are sides. C is the angle opposite side c. the Law of Cosines (also called the Cosine Rule) says:

www.mathsisfun.com

Be sure to note the section with the blue subheading: "Versions for a, b and c". You've been given the measurement for angle A only; you need to use the form containing cos(A).

EDIT: There seems to be an issue with the triangle as labeled. I'd found a solution using those measurements but was unable to confirm it, so that triangle might not exist. I was able to confirm a different solution, after changing 61º to 64º. (If we use 61º, then I think one of the given side measurements might need to change.)

$\;$

 

[Otis]

I asked someone to check using the given measurements, and they confirmed their answer (which matches my original answer). I need to go back to the drawing board (after my brain cools down).



$\;$

 

[Otis]

I don't know what happend to the OP. Here's one method.

Law of Cosines (using the known angle):

a^2 = b^2 + c^2 - 2∙b∙c∙cos(A)

where a=24, b=12 and A=61º

576 = 144 + c^2 - 2(12)(c)(0.8746)

c^2 - 11.6354∙c - 432 = 0

Solve for positive c, using the Quadratic Formula:

c = 27.4012

---

My issue before involved confirmation using sin(A)/a=sin(C)/c, to find C and then B. That did not work.

I was able to confirm c=27.4012, using sin(A)/a=sin(B)/b, to find B first and then C.

(I ought to have tried that alternate approach, before wrongly concluding a measurement issue.)



$\;$

 

[Steven G]

I asked someone to check using the given measurements, and they confirmed their solution (which matches my original solution). I need to go back to the drawing board (after my brain cools down).



$\;$

Sitting in a dark corner usually cools down the brain.

 

[Steven G]

My issue before involved confirmation using sin(A)/a=sin(C)/c, to find C and then B. That did not work.

I was able to confirm c=27.4012, using sin(A)/a=sin(B)/b, to find B first and then C.

(I ought to have tried that alternate approach, before wrongly concluding a measurement issue.)



$\;$

I too thought to use the law of sines 1st to avoid a quadratic equation. Why would the law of sines fail?

 

[Dr.Peterson]

I too thought to use the law of sines 1st to avoid a quadratic equation. Why would the law of sines fail?

It doesn't (though in principle, like the quadratic, it could have produced two solutions, and it's easier to forget that). But presumably the OP was told to use the law of cosines:

Hi, I need some help with this question. I have to solve for x using the cosine law. Thank you!
View attachment 33498

 

[Otis]

Why would the law of sines fail?

Oops, Steven. I hadn't meant to imply that. My effort to confirm the triangle using

sin(A)/a = sin(B)/b = sin(C)/c

did not work because I'd failed to consider the possibility that angle B or C might be obtuse. (I wish I could blame the op diagram for that, but I've been fooled by disproportionate diagrams before, so I ought to have known better. More likely, it was complacency on my part.)

Finding B first leads to C = 93.0676º (correct, obtuse angle)

But I'd solved for C first, getting arcsin(0.9985) = 86.9084º (incorrect), failing to consider a potential last step:

C = 180º - 86.9084º = 93.0676º

By the way, I've already spent my time in the corner for that goof, so don't even thinkaboutit!

?

 

[Dr.Peterson]

But I'd solved for C first, getting arcsin(0.9985) = 86.9084º (incorrect), failing to consider a potential last step:

C = 180º - 86.9084º = 93.0676º

Interestingly, that's exactly what I had in mind when I wrote

It doesn't (though in principle, like the quadratic, it could have produced two solutions, and it's easier to forget that).

I usually recommend using sines for a triangle like this (SSA), but the cosine approach does have this in its favor.

I think that particular corner is well-populated ...