Some problems with calculating the surface area of a rectangular pyramid.

[Thadriel]

I saw this on my niece's homework (she's in middle school), and I can't seem to get a surface area that matches all the online rectangular pyramid surface area calculators.

Anyway, here are the dimensions of the pyramid:

The rectangular base: 8 cm by 2 cm.

The two larger triangles: 8 cm across, with a "midpoint height" of 9 cm (that is, if you draw a line on the surface of the triangle from the midpoint of the 8 cm side to the opposite vertex, that length is 9 cm).

The two smaller triangles: 2 cm across, with a "midpoint height" of 9 cm (if you draw a line on the surface of triangle from the midpoint of the 2 cm side to the opposite vertex, the length is 9 cm).


Here is an image:

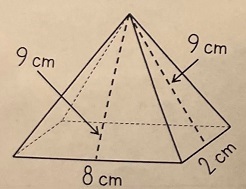
So, what I did was just calculate the surface area of the four triangles, and the surface area of the base, and add it all together.

Bigger triangle: (1/2) * 8 cm * 9 cm = 36 cm²

Smaller triangle: (1/2) * 2 cm * 9 cm = 9 cm²

Area of base: 8*2 = 16 cm²


Since there are two of each type of triangle, the total area should be:

2 * 36 cm² + 2 * 9 cm² + 16 cm² = 106 cm².
.
.

However, every rectangular pyramid calculator I put that in gives me either a surface area of about 108 cm² or about 105.99 cm², depending on which triangle I use to calculate the perpendicular height (altitude) — which I calculate as EITHER the square root of {(slant height)² - (width/2)²}, which is about sqrt(9² - 1²) = 8.944 cm, or {(slant height)² - (length/2)²}, which is about sqrt(9² - 2²) = 8.78 cm

The only way I get a matching answer with what I did above (calculating the area of each triangle and the rectangular base, then adding them all up) is by using the latter height. Why is this? Is my initial value of 106 cm² incorrect?

.
.
.


Thanks for this reading this convoluted post, and any insight you have.

 

[Dr.Peterson]

At least part of the problem is that the figure is impossible!



If you calculate the height using the two right triangles I've added, you get both $\sqrt{9^2-1^2}=\sqrt{80}\approx 8.944$ and $\sqrt{9^2-4^2}=\sqrt{65}\approx 8.062$, just as you say. (That's assuming the apex is intended to be directly above the center of the base, so that the triangles on opposite sides are the same.) The data are inconsistent.

The problem is bad! You can't find the surface area of a non-existent pyramid. Your only mistake was in not crying foul when you saw that you could find two different heights.

Now, I'd be interested to see an image of the entire problem, to make sure there is nothing else they've said that might change things.

 

[Thadriel]

That's actually what I was thinking, but I assumed there may be some space curvature going on (hehe just kidding).



That image is all there is to the problem. It just says "Find the surface area of each pyramid below. Make sure you show your work." There is a space for the student to write the area of the base and the area of the triangles. But otherwise, that's it.



So, I guess my next problem is less about math and more about diplomacy: how do we inform the teacher that this problem is ludicrous? Or should my niece just do as I did in the first part of my post, and calculate the surface areas of the triangles and base and then add them all together, and only complain if the teacher marks it wrong?

 

[The Highlander]

That's actually what I was thinking, but I assumed there may be some space curvature going on (hehe just kidding).



That image is all there is to the problem. It just says "Find the surface area of each pyramid below. Make sure you show your work." There is a space for the student to write the area of the base and the area of the triangles. But otherwise, that's it.



So, I guess my next problem is less about math and more about diplomacy: how do we inform the teacher that this problem is ludicrous? Or should my niece just do as I did in the first part of my post, and calculate the surface areas of the triangles and base and then add them all together, and only complain if the teacher marks it wrong?

Dr P's analysis is absolutely correct (and saved me drawing a new sketch too! ). The figure, as shown, is not a "real" pyramid.

All your calculations are correct but you should really have cottoned on when you got two different values for the height of the pyramid, lol. Clearly, it's impossible for the height from the centre of the base to the apex to differ depending on which side you "look" at it through.

There is absolutely no need to inform the teacher about the anomaly in the question. The question was clearly designed only to test the pupils' ability to calculate the areas of rectangles & triangles (as evidenced by the fact that "There is a space for the student to write the area of the base and the area of the triangles") and that is all that is expected of the pupils (apart from totalling them up).

The author obviously wished to keep the calculations simple (using whole numbers) and could have used a square base but probably felt that all the triangles being the same dimensions made it a little bit 'too easy' for the pupils; using the rectangular base then gives them a lit bit of extra practice in doing the area calcs. S/he would not care a jot that this made the object an impossible solid; just wants the kids to do a wee bit more work! (A commendable approach IMNSHO, as a Maths teacher. )

Your final conclusion is the correct approach: just prompt your niece to adopt your original method (unless she's already done so).

Her teacher will not mark it wrong (unless s/he has visited the same websites as you! )

 

[Thadriel]

What threw me off about the triangles with two equal sides was the fact that I forgot they were right triangles. But since you guys are here, I'll just ask:

Per the law of cosines, it appears that length c is also dependent on the angle between between a and b. I'm assuming that, for two triangles abc and a₁b₁c₁, you can have a = a₁, b = b₁, and c ≠ c₁, so long as the angle γ ≠ γ₁.

Is that right?


Anyway, it's been so long since I messed with geometry or trigonometry, and they were never my strong suites anyway. So I appreciate the help.

 

[The Highlander]

Dammit! I'd already started my sketch when Dr P posted his and I hate leaving things unfinished!
Making the figure a 'real' rectangular pyramid means the altitudes of the larger & smaller triangular faces cannot be the same and that just makes the area calcs messy for the kids!

The new (red) dimensions shown are only correct to 2 d.p., ofc.

 

[Dr.Peterson]

There is absolutely no need to inform the teacher about the anomaly in the question. The question was clearly designed only to test the pupils' ability to calculate the areas of rectangles & triangles (as evidenced by the fact that "There is a space for the student to write the area of the base and the area of the triangles") and that is all that is expected of the pupils (apart from totalling them up).

The author obviously wished to keep the calculations simple (using whole numbers) and could have used a square base but probably felt that all the triangles being the same dimensions made it a little bit 'too easy' for the pupils; using the rectangular base then gives them a lit bit of extra practice in doing the area calcs. S/he would not care a jot that this made the object an impossible solid; just wants the kids to do a wee bit more work! (A commendable approach IMNSHO, as a Maths teacher. )

I'm going to disagree a little. I agree on what was expected, but not on what should be expected.

There's probably no need to make a fuss over the bad problem; but I wouldn't commend the teacher at all. Students should not be expected to do exactly as they were taught, but to think for themselves. If a student should discover that the figure is inconsistent, that student should be given extra credit and a commendation for going beyond.

A thoughtful student (even one who hasn't learned the Pythagorean Theorem) can easily realize that the two slant heights wouldn't be the same, and ask questions. I would hope the teacher would use that as a learning opportunity, not a challenge to their authority, as some might.

And therefore, a teacher (or textbook author?) shouldn't give problems that can't be looked at too closely! One of my pet peeves is problems that are easy for unthinking students, but frustrating for the smart ones who try to take shortcuts or to check details, and find that they don't work.

So, I guess my next problem is less about math and more about diplomacy: how do we inform the teacher that this problem is ludicrous? Or should my niece just do as I did in the first part of my post, and calculate the surface areas of the triangles and base and then add them all together, and only complain if the teacher marks it wrong?

I'd agree with the latter. The problem isn't really ludicrous, just erroneous. But, especially if the problem is from a published source and not the teacher's fault, I might want to let the error be known so they could eventually correct it.

 

[Thadriel]

Dammit! I'd already started my sketch when Dr P posted his and I hate leaving things unfinished!
Making the figure a 'real' rectangular pyramid means the altitudes of the larger & smaller triangular faces cannot be the same and that just makes the area calcs messy for the kids!

The new (red) dimensions shown are only correct to 2 d.p., ofc.

While I understand why the teacher (or textbook author) might have wanted easy whole numbers, as you pointed out, I am a bit concerned that those very adept at visual, abstract thinking might have their development of an amazing talent thwarted at an early age. Maybe their intuition tells them this shape is impossible, but the problem says it isn't, so they are confused about their instinct and don't let it grow.

Granted, that's probably one kid in a million, if any, but I do wonder about it.

 

[The Highlander]

I'm going to disagree a little. I agree on what was expected, but not on what should be expected.

There's probably no need to make a fuss over the bad problem; but I wouldn't commend the teacher at all. Students should not be expected to do exactly as they were taught, but to think for themselves. If a student should discover that the figure is inconsistent, that student should be given extra credit and a commendation for going beyond.

A thoughtful student (even one who hasn't learned the Pythagorean Theorem) can easily realize that the two slant heights wouldn't be the same, and ask questions. I would hope the teacher would use that as a learning opportunity, not a challenge to their authority, as some might.

And therefore, a teacher (or textbook author?) shouldn't give problems that can't be looked at too closely! One of my pet peeves is problems that are easy for unthinking students, but frustrating for the smart ones who try to take shortcuts or to check details, and find that they don't work.


I'd agree with the latter. The problem isn't really ludicrous, just erroneous. But, especially if the problem is from a published source and not the teacher's fault, I might want to let the error be known so they could eventually correct it.

Afraid I can only to agree to disagree on this one!

I'm not terribly familiar with the American (I presume) education system but I believe "middle school" implies 11 year-olds? The question is clearly set at that level, for kids who are just learning about calculating the areas of simple, regular, plane figures. (Pythagoras would be no more than some dead Greek to them! Or a flying horse? )

The rectangular base means they have to calculate the areas of two triangles instead of just one (if the base were square), ie: extra practice.
Trying to come up with a rectangular base where the face altitudes were still whole numbers would involve unjustifiable effort (if that's even possible. I'm not going to spend any time pondering that, lol, and I'm sure the question setters didn't either!)

This problem is doubtless one of many on a sheet (or page of a book) intended to give practice solely in calculating areas for pupils who are just acquiring that skill. I would very much doubt that the OP's niece's teacher came up with it them self.

 

[The Highlander]

While I understand why the teacher (or textbook author) might have wanted easy whole numbers, as you pointed out, I am a bit concerned that those very adept at visual, abstract thinking might have their development of an amazing talent thwarted at an early age. Maybe their intuition tells them this shape is impossible, but the problem says it isn't, so they are confused about their instinct and don't let it grow.

Granted, that's probably one kid in a million, if any, but I do wonder about it.

That was probably you when you were eleven.

 

[The Highlander]

What threw me off about the triangles with two equal sides was the fact that I forgot they were right triangles. But since you guys are here, I'll just ask:

Per the law of cosines, it appears that length c is also dependent on the angle between between a and b. I'm assuming that, for two triangles abc and a₁b₁c₁, you can have a = a₁, b = b₁, and c ≠ c₁, so long as the angle γ ≠ γ₁.

Is that right?


Anyway, it's been so long since I messed with geometry or trigonometry, and they were never my strong suites anyway. So I appreciate the help.

If I understand your question correctly (I'm not sure I even understand English properly at this time of night! ) then, without recourse to any Trigonometry whatsoever, what you say is "right".

Have a look at the diagram (below) and consider what happens to c as you vary the angle between a & b (simple Geometry but, ofc, the cosine rule would still apply; who needs it! ).

 

[Thadriel]

If I understand your question correctly (I'm not sure I even understand English properly at this time of night! ) then, without recourse to any Trigonometry whatsoever, what you say is "right".

Have a look at the diagram (below) and consider what happens to c as you vary the angle between a & b (simple Geometry but, ofc, the cosine rule would still apply; who needs it! ).

I'm thinking of someone opening their mouth ?. Or opening a pair of scissors. Of course that has to be true, otherwise scissor legs would grow when you opened them ?.

You know it's weird, I have such terrible intuition for geometry, I never memorized the unit circle, and the only trig identities I know by heart are tan(x)= sin(x)/cos(x), and the Pythagorean identity, but somehow I still got pretty far in math in college, including passing some upper division undergrad math classes (the transition to higher math class, Abstract Algebra, and diff EQ II). How I managed to do that, I'll never know. Incidentally, I dropped college geometry after about three weeks in. ?

 

[The Highlander]

I'm thinking of someone opening their mouth ?. Or opening a pair of scissors. Of course that has to be true, otherwise scissor legs would grow when you opened them ?.

You know it's weird, I have such terrible intuition for geometry, I never memorized the unit circle, and the only trig identities I know by heart are tan(x)= sin(x)/cos(x), and the Pythagorean identity, but somehow I still got pretty far in math in college, including passing some upper division undergrad math classes (the transition to higher math class, Abstract Algebra, and diff EQ II). How I managed to do that, I'll never know. Incidentally, I dropped college geometry after about three weeks in. ?

Aww, what a shame! I feel certain you might have enjoyed it immensely; with a mind like yours, think of the fun you could have had asking your lecturers all kinds of abstruse questions. ?

 

[JeffM]

I am extremely dubious that (a) it is pedagogically sound to give a
child a problem that is logically inconsistent, whether or not most children will see the inconsistency, and (b) whether most teachers in U.S. middle schools can understand what the logical issue with this problem even is.

The university where I was an undergraduate (Columbia) carefully separated those getting an education degree (at Columbia Teachers College) from the students being rigorously trained at Columbia and Barnard colleges. Unlike the situation in France, where L’Ecole Normale is one of the elite schools like L’Ecole Polytechnique, the US degree in education is not designed to select and train great minds. My wife eventually refused to let me attend parent-teacher conferences because my annoyance at the incapacity of teachers to deal with the material allegedly being taught was so obvious. I was very rude to a third-grade teacher, who at the end of one such parent-teacher session pointed at Venus in the evening sky and babbled about the beauty of the stars an infinite distance away. It is criminal to allow such people to infect the minds of the innocent.

 

[Steven G]

That's actually what I was thinking, but I assumed there may be some space curvature going on (hehe just kidding).



That image is all there is to the problem. It just says "Find the surface area of each pyramid below. Make sure you show your work." There is a space for the student to write the area of the base and the area of the triangles. But otherwise, that's it.



So, I guess my next problem is less about math and more about diplomacy: how do we inform the teacher that this problem is ludicrous? Or should my niece just do as I did in the first part of my post, and calculate the surface areas of the triangles and base and then add them all together, and only complain if the teacher marks it wrong?

I think that your daughter should state that the problem can't be done. I personally did not see any problem. However in the end, the pyramid given does not exist. Students should learn to verify answers by checking everything (something that I failed to do). Imagine if this result was needed to be known for a space shuttle and the answered you got was used! The shuttle could explode.

 

[Deleted member 4993]

I think that your daughter should state that the problem can't be done. I personally did not see any problem. However in the end, the pyramid given does not exist. Students should learn to verify answers by checking everything (something that I failed to do). Imagine if this result was needed to be known for a space shuttle and the answered you got was used! The shuttle could explode.

Really not relevant - but it is niece's HW not daughter's.

 

[The Highlander]

I'm thinking of someone opening their mouth ?. Or opening a pair of scissors. Of course that has to be true, otherwise scissor legs would grow when you opened them ?.

You know it's weird, I have such terrible intuition for geometry, I never memorized the unit circle, and the only trig identities I know by heart are tan(x)= sin(x)/cos(x), and the Pythagorean identity, but somehow I still got pretty far in math in college, including passing some upper division undergrad math classes (the transition to higher math class, Abstract Algebra, and diff EQ II). How I managed to do that, I'll never know. Incidentally, I dropped college geometry after about three weeks in. ?

Further to #13... You see the trouble you've caused in here, Thadriel, over a dumb question for an eleven-year-old's homework? lmao. ??

 

[Thadriel]

I am extremely dubious that (a) it is pedagogically sound to give a
child a problem that is logically inconsistent, whether or not most children will see the inconsistency, and (b) whether most teachers in U.S. middle schools can understand what the logical issue with this problem even is.

The university where I was an undergraduate (Columbia) carefully separated those getting an education degree (at Columbia Teachers College) from the students being rigorously trained at Columbia and Barnard colleges. Unlike the situation in France, where L’Ecole Normale is one of the elite schools like L’Ecole Polytechnique, the US degree in education is not designed to select and train great minds. My wife eventually refused to let me attend parent-teacher conferences because my annoyance at the incapacity of teachers to deal with the material allegedly being taught was so obvious. I was very rude to a third-grade teacher, who at the end of one such parent-teacher session pointed at Venus in the evening sky and babbled about the beauty of the stars an infinite distance away. It is criminal to allow such people to infect the minds of the innocent.

Incidentally my girlfriend went there for astronomy. She once shared an elevator with Brian Greene.

Further to #13... You see the trouble you've caused in here, Thadriel, over a dumb question for an eleven-year-old's homework? lmao. ??

I appear to be an agent of chaos.

I think that your daughter should state that the problem can't be done. I personally did not see any problem. However in the end, the pyramid given does not exist. Students should learn to verify answers by checking everything (something that I failed to do). Imagine if this result was needed to be known for a space shuttle and the answered you got was used! The shuttle could explode.

Was there not an incident where a craft was lost due to a simple unit error? Yes, that's right, a 125 million dollar (U.S.) piece of equipment was lost because an engineering group used English units while most of the team used metric.

https://www.simscale.com/blog/2017/12/nasa-mars-climate-orbiter-metric/

How humiliating.

 

[The Highlander]

Incidentally my girlfriend went there for astronomy. She once shared an elevator with Brian Greene.

I appear to be an agent of chaos.

Was there not an incident where a craft was lost due to a simple unit error? Yes, that's right, a 125 million dollar (U.S.) piece of equipment was lost because an engineering group used English units while most of the team used metric.

https://www.simscale.com/blog/2017/12/nasa-mars-climate-orbiter-metric/

How humiliating.

Re: "an engineering group used English units while most of the team used metric."
I hold the English to be guilty of many things (not least the continuing subjugation of my (& God's) own country) but I can't agree that it's fair to accuse them of persisting in the use of the Imperial (not English) system of units!
It seems to me that it is (you?) Americans who persist in using gallons, ft., lbs. & °F, etc. ?

 

[Otis]

How humiliating.

Oh, that's old news. We've quite outdone ourselves, since then!