never_lose
New member
- Joined
- Jul 9, 2011
- Messages
- 7
Sketch the region Ω and change the order of integration.
∫14∫x2xf(x,y)dydx
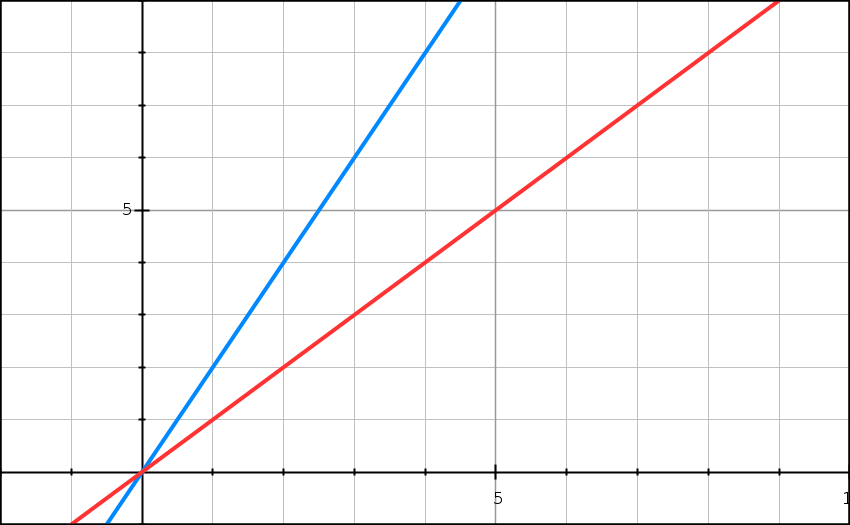
This is my sketch, except there should be vertical asymptotes at x = 1 and x = 4. Ω would be the region between x = 1 and x = 4 and the two lines.
When I switch, y should be in terms of x... so I solved for x from these two equations: y=2x and y=x (which are the equations for the red and blue lines)
x=2y x=y.
x should be expressed in terms of y so I use x=21 x=y.
I then get ∫14∫yy/2f(x,y)dxdy.
But the answer should be ∫12∫1yf(x,y)dxdy+∫24∫y/2yf(x,y)dxdy+∫48∫y/24f(x,y)dxdy.
I'm not getting what is happening. How are the integrals getting split up into separate parts?
∫14∫x2xf(x,y)dydx
This is my sketch, except there should be vertical asymptotes at x = 1 and x = 4. Ω would be the region between x = 1 and x = 4 and the two lines.
When I switch, y should be in terms of x... so I solved for x from these two equations: y=2x and y=x (which are the equations for the red and blue lines)
x=2y x=y.
x should be expressed in terms of y so I use x=21 x=y.
I then get ∫14∫yy/2f(x,y)dxdy.
But the answer should be ∫12∫1yf(x,y)dxdy+∫24∫y/2yf(x,y)dxdy+∫48∫y/24f(x,y)dxdy.
I'm not getting what is happening. How are the integrals getting split up into separate parts?

