In certain deep parts of oceans, the pressure of sea water,
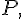 in pounds per square foot, at a depth of
in pounds per square foot, at a depth of
 feet below the surface, is given by the following equation:
feet below the surface, is given by the following equation:

If a scientific team uses special equipment to measures the pressure under water and finds it to be 562 pounds per square foot, at what depth is the team making their measurements?
Answer: The team is measuring at ____ feet below the surface.
If a scientific team uses special equipment to measures the pressure under water and finds it to be 562 pounds per square foot, at what depth is the team making their measurements?
Answer: The team is measuring at ____ feet below the surface.
