How many litres of paraffin will a similar drum 30 cm high hold?
- Thread starter chijioke
- Start date
Again think ... what does SIMILAR solid mean?
I am still thinking of volumes.
Instead, look up the meaning through Google (or otherwise).
I have searched but couldn't find anything reasonable to help my understanding.
topsquark
Senior Member
- Joined
- Aug 27, 2012
- Messages
- 2,269
Let's go sideways a moment. Say we have a square with an area of 9 m^2 and a side of 3 m. A similar square has a side of 2 m. What is the area of the smaller square?
Let's forget about similarity for a moment. The smaller square has a side of 2 m, so it's area is 4 m^2, right?
Look at the following:
[imath]\dfrac{ \text{area of big square} }{ \text{area of little square} } = \dfrac{ \text{9 m^2} }{ \text{4 m^2} } = \left ( \dfrac{3}{2} \right )^2[/imath]
(Sorry, I can't figure out where I'm doing the LaTeX wrong.)
The area goes as the square of the ratio of the sides. What do you think happens with the volume?
-Dan
Dr.Peterson
Elite Member
- Joined
- Nov 12, 2017
- Messages
- 16,088
[imath]\dfrac{ \text{area of big square} }{ \text{area of little square} } = \dfrac{ 9\text{ m}^2 }{ 4\text{ m}^2 } = \left ( \dfrac{3}{2} \right )^2[/imath]
(Somehow it doesn't like "^" inside text.)
Similar areas and volumes - Using similarity - National 5 Maths Revision - BBC Bitesize
Calculate the size of a missing length, area or volume on a similar figure using the enlargement/reduction scale factor as part of National 5 Maths.
www.bbc.co.uk
The volume goes as the cube of the sides.
The Highlander
Full Member
- Joined
- Feb 18, 2022
- Messages
- 937
No, (since this is a cylinder, not a cube) consider the ratios: If a line is 2m long then a 4m line is 2 times as long and an 8m line is 4 times as long but if a square has 2m sides it's area is 4m² and a square with 4m sides has and an area of 16m² (not 8m²) because areas vary by the square of their ratios.
So how do you think volumes will vary?
Last edited:
The Highlander
Full Member
- Joined
- Feb 18, 2022
- Messages
- 937
And what is the ratio of the sides?...
The Highlander
Full Member
- Joined
- Feb 18, 2022
- Messages
- 937
The key word in your question is "similar" which means that all the dimensions in each drum will have the same ratio (ie; not only the sides will be shorter but the area of the base will will be smaller too).
Their volumes will, therefore, be related through that ratio (in a particular way).
You are nearly there. ?
Their volumes will, therefore, be related through that ratio (in a particular way).
You are nearly there. ?
Really - please think before you ask the question?
The amount (volume) that a cylinder can hold is directly proportional to the volume .
Amount of tea a cup can hold is directly proportional to the "internal" volume of the cup. If cup A has twice the volume of cup B - then cup A will hold twice the amount of tea compared to cup B.
The Highlander
Full Member
- Joined
- Feb 18, 2022
- Messages
- 937
You still seem to be struggling with this even though it is actually a very simple problem to deal with so I will give you a very simple example that should help you reach the correct answer.
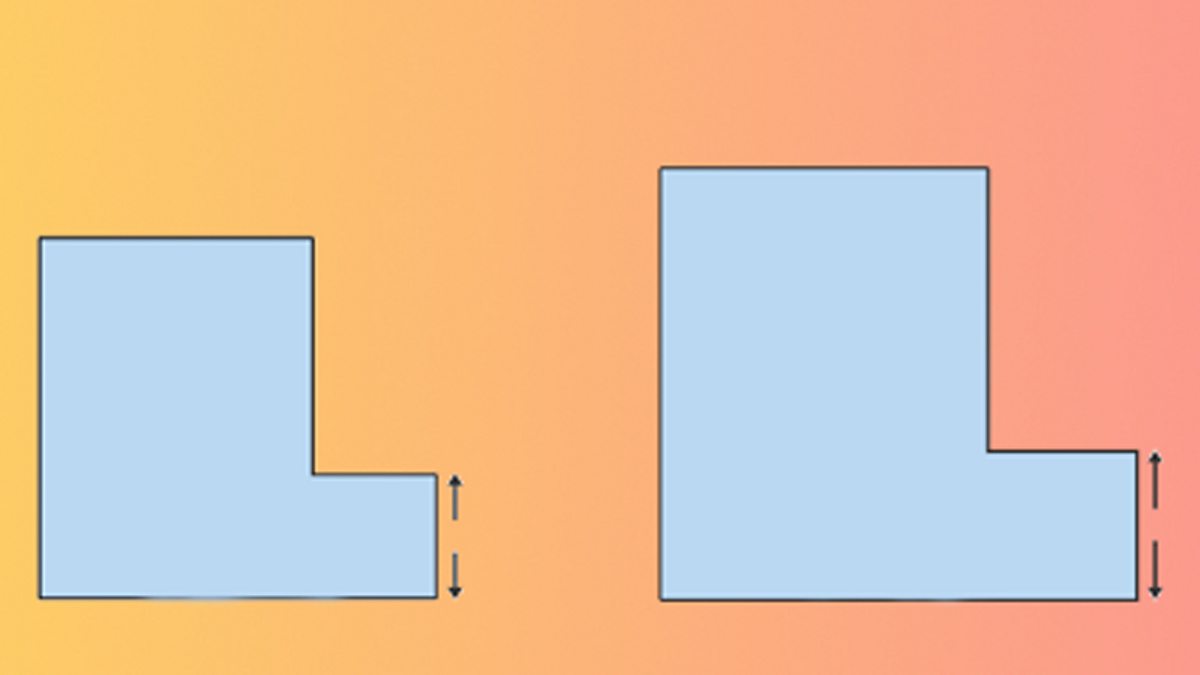
Below are two containers that you are told are similar (which we have already stressed means that all their dimensions are in the same ratio), therefore, it doesn’t matter what shape they are, we can work out relative volumes if we know just that ratio.
Since these containers just happen to be cubic boxes (for the purposes of this explanation) it is easy to calculate the actual volume of each box, so I have shown those volume calculations on the diagram.
So what is the ratio of the two sides given?
Well it is 3:4.5 which = 2:3 = \(\displaystyle \frac{2}{3}\) (in fractional form)
Now we have repeatedly hinted at (and I thought you had grasped this) that the Volume relationship is cubic (just as the Area relationship was square)
Thus the relationship of their volumes is \(\displaystyle \left(\frac{2}{3}\right)^3=\frac{8}{27}\)
And \(\displaystyle \frac{8}{27}\) × 91.125 = ??? (Use your calculator)
Can you now get the answer to the original problem (without further ado)?
Maybe this is the solution you are expecting me to obtain. My greatest challenge here is how do I know whether am to use linear, area or volume factor in solving this kind of problem?
The Highlander
Full Member
- Joined
- Feb 18, 2022
- Messages
- 937
Yes, 3.2 litres is the correct answer. (Though I don't understand why you felt the need to invert the ratio when I had already shown you the 'simpler' approach. ?)
It tells you what you are dealing with in the problem(s)!
Litres (or m³ or cm³ or the like) are measures of Volume, while Areas will be in square units (eg: m², cm², ft², in² etc.) and Lengths are just inches, feet, millimetres, centimetres, kilometres, etc.
Read the questions carefully and think about these things.
Sorry! I saw it, but never really took my time go through it. If decide to use [math]\frac{8}{27}×10.8[/math], I will still get the 3.2 litres- meaning that 10.8 litres was decreased in the ratio [math]8:27[/math]. That's not bad anyway.
Or do we just say anytime before solid shape is involved we should be talking about solid shape? I am asking this because cylinder is a three dimensional shape.
The Highlander
Full Member
- Joined
- Feb 18, 2022
- Messages
- 937
What "we" do is we think about things.
Consider carefully the wording of problems, look for important terms (like 'similar') that often tell you a lot more than a single word usually does and sort out in your mind what it is you are dealing with before you rush into any calculations. ?
Okay.??
A classic way to solve any problem of this type.
\(\displaystyle \frac{h}{H} = \frac{d}{D}\)
\(\displaystyle h:\) height of the small cylinder.
\(\displaystyle H:\) height of the big cylinder.
\(\displaystyle d:\) diameter of the small cylinder
\(\displaystyle D:\) diameter of the big cylinder
We are given the height and the volume of the big cylinder, so we can find its diameter \(\displaystyle D\).
\(\displaystyle V = \pi R^2 H = \pi \left(\frac{D}{2}\right)^2H\)
\(\displaystyle D = \sqrt{\frac{4V}{\pi H}}\)
Back to our classic formula.
\(\displaystyle \frac{h}{H} = \frac{d}{D} = \frac{d}{\sqrt{\frac{4V}{\pi H}}}\)
Now, we can find the diameter of the small cylinder.
\(\displaystyle d = \frac{h\sqrt{\frac{4V}{\pi H}}}{H} = \frac{0.30\sqrt{\frac{4 \times 10.8}{\pi \times 0.45}}}{0.45} \approx 3.68527\) m
Finally, we can find the volume of the small cylinder.
\(\displaystyle V_S = \pi r^2 h = \pi \left(\frac{d}{2}\right)^2 h = \pi \left(\frac{3.68527}{2}\right)^2 \times 0.30 = 3.2\) litres
\(\displaystyle \frac{h}{H} = \frac{d}{D}\)
\(\displaystyle h:\) height of the small cylinder.
\(\displaystyle H:\) height of the big cylinder.
\(\displaystyle d:\) diameter of the small cylinder
\(\displaystyle D:\) diameter of the big cylinder
We are given the height and the volume of the big cylinder, so we can find its diameter \(\displaystyle D\).
\(\displaystyle V = \pi R^2 H = \pi \left(\frac{D}{2}\right)^2H\)
\(\displaystyle D = \sqrt{\frac{4V}{\pi H}}\)
Back to our classic formula.
\(\displaystyle \frac{h}{H} = \frac{d}{D} = \frac{d}{\sqrt{\frac{4V}{\pi H}}}\)
Now, we can find the diameter of the small cylinder.
\(\displaystyle d = \frac{h\sqrt{\frac{4V}{\pi H}}}{H} = \frac{0.30\sqrt{\frac{4 \times 10.8}{\pi \times 0.45}}}{0.45} \approx 3.68527\) m
Finally, we can find the volume of the small cylinder.
\(\displaystyle V_S = \pi r^2 h = \pi \left(\frac{d}{2}\right)^2 h = \pi \left(\frac{3.68527}{2}\right)^2 \times 0.30 = 3.2\) litres